MOLA Random Vibration Analysis
If you are interested in learning some basics about random vibration analysis, then feel free to read on or make the jump to the fourth link below. If you are only interested in MOLA-specific information, then click on your area of interest in the list. If you have any questions, then you can contact me at the e-mail address located at the bottom of this page.
When random analysis or testing is performed, an input spec is needed. It gives the frequency range, e.g., 20-2000Hz, and it gives the Power Spectral Density (PSD) level, i.e., the magnitude of the random input. This spec tells the PSD level of random vibration that was input to the MOLA instrument finite element model.
In this set of pages you will find the response charts of the following MOLA components:
- Laser Housing
- Telescope Assembly: Secondary Mirror; Solar Shield Doghouse
- Detector Housing
- Electronics Box
- Titanium Support Tube
- Feet/Support Panel interface
An exploded view of the model is available to identify the components.
In this set of pages you will find the force response chart of the MOLA instrument. This chart shows the force acting at the spacecraft/instrument interface that is caused by the random vibration of the MOLA instrument.
What is Random Vibration?
Random vibration is exactly what the name describes: vibrations that occur randomly. Driving down the road makes your car vibrate. You never know when you will hit a bump in the road because they occur randomly. This is different from the vibration caused by your engine or tires rotating in regular cycles.
The measurement of vibration is frequency or cycles per second, which is given in Hertz (Hz). In regular, cyclic vibrational analysis, only one frequency at a time is of interest. We may sweep through a range of frequencies, as in Frequency Response Analysis, but we are really only interested in the response at one frequency, such as at a natural frequency.
In random vibration however, you can have all frequencies occuring simultaneously. Because of this, random vibration analysis is usually performed over a large range of frequencies, say from 20 to 2000 Hz (see the chart below). We are not looking at a specific frequency, specific moment in time or specific anything else; we are statistically looking at a structure's response to a given random vibrational environment. Certainly we want to know if there are any frequencies that cause a large random response at any natural frequencies, but mostly we want to know the overall response of the structure.
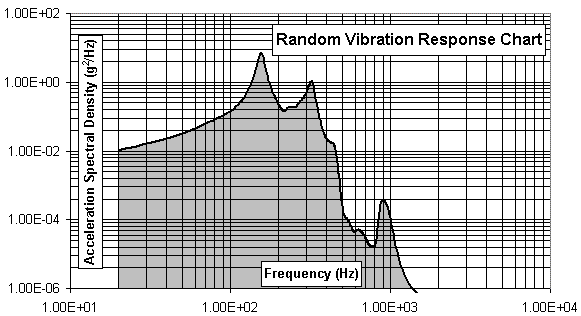Think of it this way: Often, we like to measure something over a period of time, such as how many cars go through an intersection. Then, we can average the number of cars over the length of an hour or over a number of days. Because we're doing this over a period of time, we call this the time domain. You all know that probability and statistics are used for such investigations. In fact, these results would be called a Probability Density.
Random vibration analysis looks at random accelerations or forces over a range of frequencies, which we call the frequency domain. (These random inputs are merely sustaned over a period of time, but are not time-dependent; i.e., the longer the period of time, the better the statistical sampling in the frequency domain.) The range of frequencies is called a spectrum. Therefore, we call these results a Spectral Density. Generically, we use Power Spectral Density*, although this isn't exactly correct. If we are looking at accelerations, we use Acceleration Spectral Density (ASD), and for forces, we use Force Spectral Density (FSD).
The chart shows the area under the curve as gray. This area is actually what we are interested in. If we take the square root of this area, we then have the root mean square value of the acceleration, better known as Grms. This value is what we use in our analysis calculations, for example in our stress calculations. If the area is large due to a high response, then we may have problems; likewise, if the area is small, then we have a small Grms value and we shouldn't have any problems.
Deriving any of these quantities is far beyond the scope of what I'm trying to explain here, so I won't bother. I just hope my simple explanation itself wasn't far beyond the scope of what I am trying to expalin.
* The term Power in Power Spectral Density seems to come from the fact that when random vibration measurements were taken, they were actually recorded electronically and so the power levels were used in the calculations.
Why do we care about random vibration?
Simply put, rather large accelerations can occur during random vibration events. As things vibrate back and forth, accelerations and decelerations are happening with each cycle. As you know, F=ma, so if a large acceleration occurs during random vibration, then the forces can also get very large.
What causes random vibration?
Here at NASA, there are only a few things that create random vibration that concern us: ground transportation equipment, various unmanned launch vehicles (like the Titan and the Delta rockets), and of course, the Space Shuttle . Since we've had plenty of experience with getting things into space, we know what levels these random vibrations occur at. From studying these sources, engineers have created random vibration input specifications. A random input spec is generally given either in a table or graphically.
When random vibration analysis is performed, the model is "shaken" at the point or points where the model interfaces with either the spacecraft, the launch vehicle, or at least another, much larger component--a larger mass always drives a smaller mass. If more than one point is constrained to a larger mass, then those points are rigidly connected together at one point, as is the case with MOLA. MOLA has three feet with six mounting points per foot. Thus, these 18 points are connected down to what we call the shaker point. This shaker point is where the random vibrational input is applied.
From this input, responses can be obtained at any location on the finite element model.
As I mentioned above, random vibration is merely a statistical sampling of vibrations that can occur over a range of frequencies. So what do we do if we randomly encounter a very high response during testing? This could cause unrealistically high forces to act on the instrument. Obviously we don't want to break the instrument just because we cannot be certain what will be happening during random testing.
In the past, what we did was notch the input spec where we thought we might encounter extremely high responses. That is, we looked at the frequencies where the high responses occur and we lower the input spec by the factor that the response is above the input. For example, if the response is 1.5 times higher than our upper limit, then we divide the input by 1.5 at that frequency; if the response is 2 times our limit, we divide the input by 2. The result is an input plot that literally looks like notches were cut out of it. Jumping to the notched input page will show you exactly what I mean.
Notching is good, except we determine the notching from the finite element model, which does not mirror exactly what will happen to the actual instrument. If the notch is off by a few Hz, a high response could still occur. Even though we still do notching, we also employ an even better method of safeguarding the instrument called force limiting.
Force limiting requires that force sensors be placed between the test table and the test item. Then, during random testing, a computer monitors the forces going into the instrument. If the computer sees that the forces are rising toward a predetermined level, it will dynamically reduce the random input so that the upper force limit is not reached. Force limiting together with input spec notching give us a pretty good feeling that our instrument will be safe during testing.
Back to the Top of the Page.
Back to the MOLA Mechanical Analysis Page.
This page is maintained by Ryan Simmons, at Ryan.Simmons@nasa.gov.
This page was last updated on January 22, 1996.